Research
Correlated Many-Body Systems and Electronic Structure of Solids
- Theory of interacting electron systems
- Topological states of matter
- Realistic calculations of novel and funtional materials
- Nanoscopic systems
- Computational methods for strongly correlated fermions *)
- Electron-phonon interaction and high-Tc superconductors
*) to use the "w2dynamics" code, please contact Prof. Giorgio Sangiovanni
Dynamical Correlations and Order in Magic-Angle Twisted Bilayer Graphene
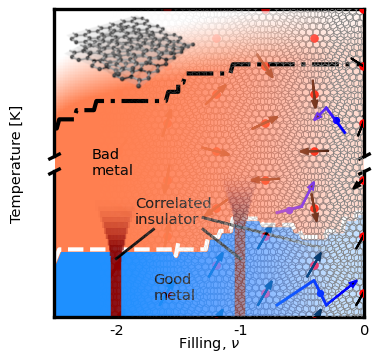
Superconductor surprises with strongly interacting electrons
Umbrella for Atoms: The First Protective Layer for 2D Quantum Materials
Mott insulators with boundary zeros
“3D glasses” for topological materials: Using hi tech to illuminate quantum mysteries
Nat. Phys. 19, 1135–1142 (2023)
Method Milestone for Quantum Physics: Rapid Test for Topological 2D Materials
Twofold van Hove singularity and origin of charge order in topological kagome superconductor CsV3Sb5
Triangular Honeycombs: Physicists design novel quantum material
Nature Communications 12, 5396 (2021)
Design and realization of topological Dirac fermions on a triangular lattice
Nature Communications 12, 5396 (2021)
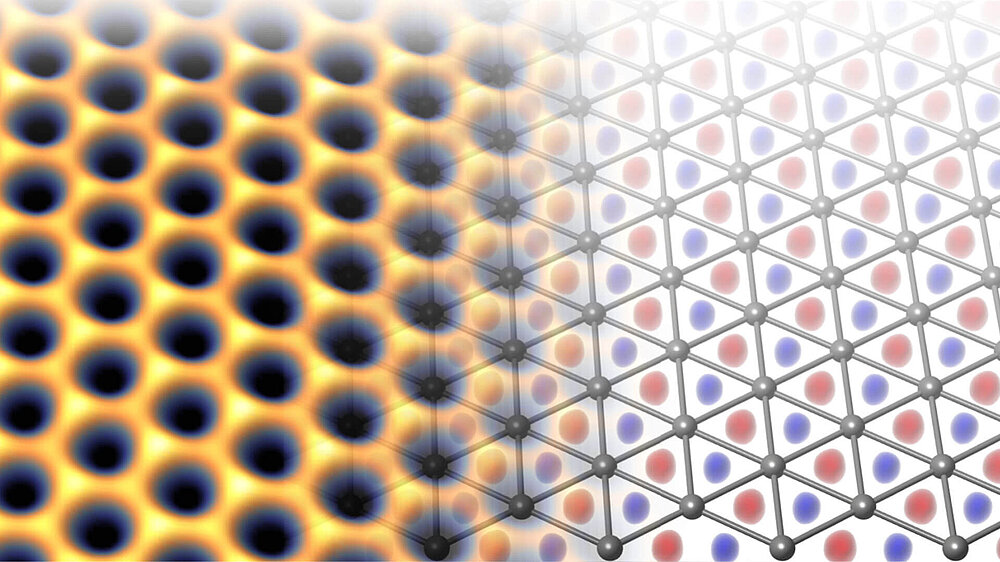
Local magnetic moments in iron and nickel at ambient and Earth’s core conditions

Some Bravais lattices have a particular geometry that can slow down the motion of Bloch electrons by pre-localization due to the band-structure properties. Another known source of electronic localization in solids is the Coulomb repulsion in partially filled d or f orbitals, which leads to the formation of local magnetic moments. The combination of these two effects is usually considered of little relevance to strongly correlated materials. Here we show that it represents, instead, the underlying physical mechanism in two of the most important ferromagnets: nickel and iron. In nickel, the van Hove singularity has an unexpected impact on the magnetism. As a result, the electron–electron scattering rate is linear in temperature, in violation of the conventional Landau theory of metals. This is true even at Earth’s core pressures, at which iron is instead a good Fermi liquid. The importance of nickel in models of geomagnetism may have therefore to be reconsidered.


